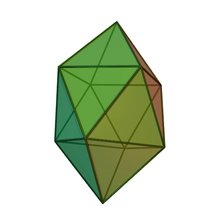
Gyroelongated square bipyramid

Gyroelongated square bipyramid
Type	Gyroelongated bipyramid, Deltahedron, Johnson J16 – J17 – J18
Faces	16 triangles
Edges	24
Vertices	10
Vertex configuration	$2\times (3^{4})+8\times (3^{5})$
Symmetry group	$D_{4d}$
Dual polyhedron	Truncated square trapezohedron
Properties	convex
Net

In geometry, the gyroelongated square bipyramid is a polyhedron with 16 triangular faces. it can be constructed from a square antiprism by attaching two equilateral square pyramids to each of its square faces. The same shape is also called hexakaidecadeltahedron, heccaidecadeltahedron, or tetrakis square antiprism; these last names mean a polyhedron with 16 triangular faces. It is an example of deltahedron, and of a Johnson solid.

The dual polyhedron of the gyroelongated square bipyramid is a square truncated trapezohedron with eight pentagons and two squares as its faces. The gyroelongated square pyramid appears in chemistry as the basis for the bicapped square antiprismatic molecular geometry, and in mathematical optimization as a solution to the Thomson problem.

Construction

Like other gyroelongated bipyramids, the gyroelongated square bipyramid can be constructed by attaching two equilateral square pyramids onto the square faces of a square antiprism; this process is known as gyroelongation. These pyramids cover each square, replacing it with four equilateral triangles, so that the resulting polyhedron has 16 equilateral triangles as its faces. A polyhedron with only equilateral triangles as faces is called a deltahedron. There are only eight different convex deltahedra, one of which is the gyroelongated square bipyramid. More generally, the convex polyhedron in which all faces are regular is the Johnson solid, and every convex deltahedron is a Johnson solid. The gyroelongated square bipyramid is numbered among the Johnson solids as $J_{17}$.

One possible system of Cartesian coordinates for the vertices of a gyroelongated square bipyramid, giving it edge length 2, is: $${\begin{aligned}\left(\pm 1,\pm 1,2^{-1/4}\right),\qquad &\left(\pm {\sqrt {2}},0,-2^{-1/4}\right),\\\left(0,\pm {\sqrt {2}},-2^{-1/4}\right),\qquad &\left(0,0,\pm \left(2^{-1/4}+{\sqrt {2}}\right)\right).\end{aligned}}$$

Properties

The surface area of a gyroelongated square bipyramid is 16 times the area of an equilateral triangle, that is: $$4{\sqrt {3}}a^{2}\approx 6.928a^{2},$$ and the volume of a gyroelongated square bipyramid is obtained by slicing it into two equilateral square pyramids and one square antiprism, and then adding their volume: $${\frac {{\sqrt {2}}+{\sqrt {4+3{\sqrt {2}}}}}{3}}a^{3}\approx 1.428a^{3}.$$

3D model of a gyroelongated square bipyramid

It has the same three-dimensional symmetry group as the square antiprism, the dihedral group of $D_{4d}$ of order 8. Its dihedral angle is similar to the gyroelongated square pyramid, by calculating the sum of the equilateral square pyramid and the square antiprism's angle in the following:

• the dihedral angle of an equilateral square pyramid between two adjacent triangles, approximately $109.47^{\circ }$
• the dihedral angle of a square antiprism between two adjacent triangles, approximately $127.55^{\circ }$
• the dihedral angle between two adjacent triangles, on the edge where an equilateral square pyramid is attached to a square antiprism, is $158.57^{\circ }$, for which by adding the dihedral angles between a square and a triangle of both the pyramid and the antiprism.

The dual polyhedron of a gyroleongated square bipyramid is the square truncated trapezohedron. It has eight pentagons and two squares.

Application

Gyroelongated square bipyramid can be visualized in the geometry of chemical compounds as the atom cluster surrounding a central atom as a polyhedron, and the compound of such cluster is the bicapped square antiprismatic molecular geometry. It has 10 vertices and 24 edges, corresponding to the closo polyhedron with $2n+2$ skeletal electrons. An example is nickel carbonyl carbide anion Ni₁₀C(CO)²⁻
₁₈, a 22 skeletal electron chemical compound with ten Ni(CO)₂ vertices and the deficiency of two carbon monoxides.

The Thomson problem concerning the minimum-energy configuration of $n$ charged particles on a sphere. The minimum solution known for $n=10$ places the points at the vertices of a gyroelongated square bipyramid, inscribed in a sphere.